________________
________________
Poor Pascal
“I’ve discovered a revolutionary new way to generate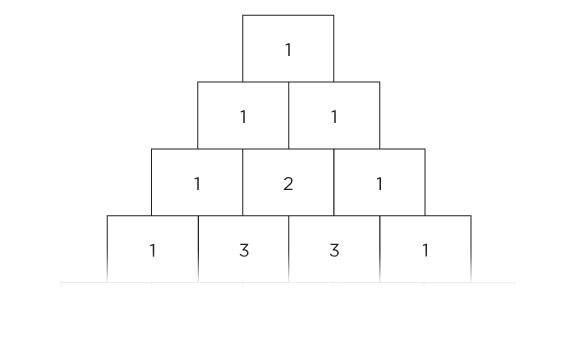
Pascal’s triangle!” crows Rascal. “This is another one of your tricks,” says a senior on the team. “No, try it,” pleads Rascal. “You make the only number in the first row, and the first and last number in all other rows, a one, as you normally would for Pascal’s triangle. Fill in every other entry by taking the product of the two numbers above it to the left and right (instead of the sum like you would usually), adding one and then dividing by the number directly above it, two rows up.” The senior starts making the triangle and finds that everything matches up after filling in a dozen entries. She exclaims, “Wow, maybe you’re on to something!”
Rascal asks: “What’s the middle entry in the third row, times the middle entry of the fifth row, times the 2,304th entry in the 4,609th row, if you keep following my rule? And why did I choose those entries?”
Rascal’s Grid
Rascal dances over to a junior and says, “I’ve got a grid of numbers for you! Get a sheet of graph paper and fill the first row (the row at the top) with ones. I call that ‘counting by zeros.’ Fill in the second row with the whole numbers starting from one: I call that ‘counting by ones.’ Fill in the third row with the odd numbers: I call that ‘counting by twos.’ Fill in the fourth row by counting by threes: 1, 4, 7, and so on. Keep filling in rows similarly, counting by one more in each successive row.”
Rascal asks: “What’s the 57th entry in the 23rd row minus the 23rd entry in the 57th row? And what does this have to do with my other problem?”
| Spread the word: | Tweet |
Solutions to week 80
Party Puzzle. For the 11th person to be the winner of the door prize, there have to be at least eleven people. So let’s see what happens with eleven people; the numbers of the people that get the red tickets are, in order, 2, 4, 6, 8, 10, 1, 5, 9, 3, 11, so the seventh person wins the prize. With twelve people, the ticket recipients are 2, 4, 6, 8, 10, 12, 3, 7, 11, …, so clearly the 11th person does not win the prize. With 13 people, however, the tickets go to people numbered 2, 4, 6, 8, 10, 12, 1, 5, 9, 13, 7, 3, so person 11 wins the door prize. Hence the fewest number of people that could have been at the party is 13.
Secret Remainder. Coach Taylor discusses this one herself: There are two ways you could attack this. If you know some interesting facts about different types of numbers, here’s how it could go. Since the square minus one was a multiple of eight, it must have been the square of an odd number. But any triangular number times eight plus one is the square of an odd number, and conversely, any odd square minus one divided by eight is a triangular number. So the secret number S is a triangular number. Every triangular number has a remainder of 0, 1, 3, or 6 when divided by nine. But since S is not a multiple of three, its remainder when divided by nine must be one.
But even if you didn’t know those facts about square and triangular numbers, continues Coach Taylor, you can still do this problem. Again, start with the observation that the starting number is the square of an odd number, so it is of the form (2n+1)² = 4n² + 4n + 1. When we subtract one and divide by eight, we get that S = (n² + n)/2, which is a whole number because either n² and n are both odd or both even, so the numerator is always even. Now clearly if n was divisible by 3, then S would also be, so n must not be divisible by three. Similarly, if n was of the form 3k+2, then S = (9k² + 12k + 4 + 3k + 2)/2 = 3(3k² + 5k + 2)/2 would be a multiple of three. Therefore, n must be of the form 3k+1, which means that S = (9k² + 6k + 1 + 3k + 1) / 2 = 9(k² + k)/2 + 1 has a remainder of one when divided by nine.
Recent Weeks
Week 80: Secret Remainder & Party Puzzle, solutions to Wing Nuts & Seven Eleven
Week 79: Wing Nuts & Seven Eleven, solutions to Polychromatic Pigs & Guest Gifts
Week 78: Polychromatic Pigs & Guest Gifts, solutions to Trial Squares & Four of Six
Week 77: Trial Squares & Four of Six, solutions to Unleash Your Inner Rectangle & Pent-up-gon
Week 76: Unleash Your Inner Rectangle & Pent-up-gon, solutions to Some Difference & In Your Prime
Links to all of the puzzles and solutions are on the Complete Varsity Math page.
Come back next week for answers and more puzzles.
[asciimathsf]
