
________________
Coach Newton says it’s always valuable to follow up on earlier problems, so today the team looks back at the recent and not-so-recent past.
________________

Further Fairness
The four teammates who shared the cookie in Edible Division last week are still amazed that as long as they cut from the center, any way they divide the perimeter equally produces pieces of equal area. This makes them curious about their next item up for baking — the Pythagorean Cake, with side lengths 3 inches, 4 inches, and 5 inches.
Is there a point on the Pythagorean Cake so that any four straight cuts from that point to the edges of the cake that divide the perimeter into four equal lengths will also divide the area of the cake into four equal portions?
Double-Crossed Training
Oddly enough, six of the team members end up in exactly the same situation as a year ago in Cross Training: on one side of a river with a boat that holds at most three of them, all needing to get to the other side. The team members can make it across individually in 1, 2, 3, 5, 8, and 13 minutes, respectively. When there are two in the boat, it takes one minute less than the slower passenger. And when there are three in the boat, it takes the same length of time as the middle passenger.
What is the smallest number of minutes needed for all six teammates to cross the river?
| Spread the word: | Tweet |
Solutions to week 60
Edible Division. Start with a solution for dividing the cookie into three pieces: clearly cutting it from the center to each vertex works, as in the leftmost diagram. Now, to divide it into four pieces with equal area, we need each piece to have 3/4 as much area as in this solution. Is there an easy way to arrange that? Sure, just make the base of one of the pieces 3/4 as big, leaving its height alone, as in the next diagram to the right. And in fact, we can make two pieces like that, as in the third diagram. And since those two pieces now take up half of the area of the cookie, if we cut what’s left into two identical pieces as in the last diagram, each piece will have a quarter of the total area. But now look, all four pieces have an equal amount of chocolate, 3/4 of the amount of the original three pieces, because the first two have 3/4 of a side each, and the last two have the remaining 1/4 from one side and 1/2 of the final side. So we’ve solved it! 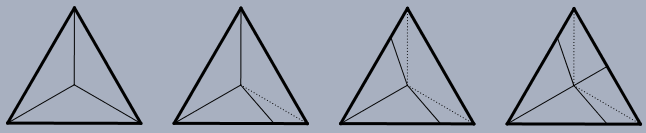
But wait, there’s more! Any way we divide the perimeter into four equal sections, if we cut to the center, we will create pieces of equal area. That’s because as shown every piece can be divided into two sections which are triangles with height equal to the original three pieces, and bases which add up to 3/4 of the original side, and so together have area equal to 3/4 of the original pieces.



Counting Cars. Each car requires four minutes to rinse and there’s only one hose. And the fastest any single car can be washed, if all four team members work on it, takes 12/4 = 3 minutes. However, if one teammate is rinsing and the other three are washing, each group takes four minutes to complete a car. So they can work perfectly in parallel, except first there needs to be (at least) one car ready to rinse. That happens fastest if all four students work on it, and then one stays with that car to rinse and the other three start washing the next car. Then just as the rinser finishes the first car, the other three will have finished the second car and the rinsing can continue. In this fashion, the total time needed is 10 × 4 minutes (for all of the rinsing) plus 3 minutes (to prepare the first car for rinsing), or 43 minutes.














