Math Monday: Paper Polyhedra
by George Hart

After mastering the five Platonic solids, there is a world of more complex models to explore. The polyhedron below consists of twelve regular pentagons and twenty (very slightly irregular) hexagons. It is made by cutting out paper polygons and taping them together on the inside. This design is often confused with the truncated icosahedron shape that is well known because of its use as a soccer ball. But this shape is the truncated rhombic triacontahedron. To see the difference, notice that there are some vertices here with three hexagons and no pentagon, but in a soccer ball there is one pentagon and two hexagons at each vertex.
 And if you become engaged in discovering the world of polyhedra, you will encounter the many additional families, including the stellated icosahedron below. Their intricacies can be quite a challenge to make from paper, especially when some components meet just at points. I made the model below over thirty years ago, starting from a template in the book Polyhedron Models by Magnus Wenninger. If you want your models to last this long, be sure to use acid-free paper.
And if you become engaged in discovering the world of polyhedra, you will encounter the many additional families, including the stellated icosahedron below. Their intricacies can be quite a challenge to make from paper, especially when some components meet just at points. I made the model below over thirty years ago, starting from a template in the book Polyhedron Models by Magnus Wenninger. If you want your models to last this long, be sure to use acid-free paper.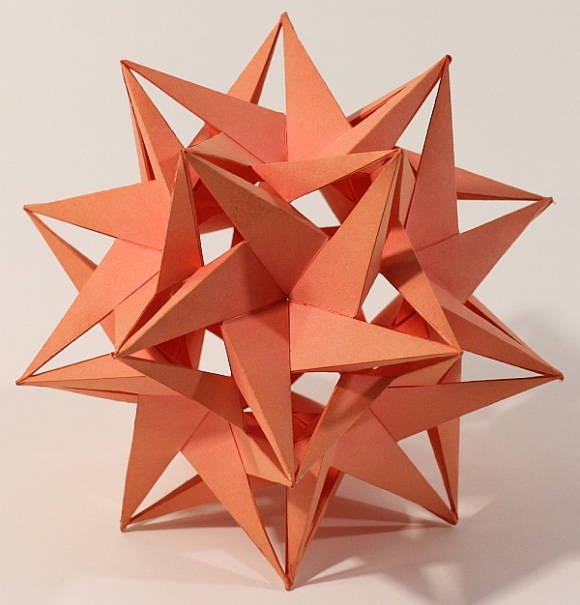
This article first appeared on Make: Online, April 30, 2012.












