Math Monday: Light Still Sticks
by Glen Whitney
What do you get with a handful of lightsticks, a bag of rubber bands, and a box brass fasteners? Well, if you follow the instructions here you get a beautiful glowing octahedron or even a radiant ”Nechushtan spindle.” Here’s what you do:
Ingredients: A bunch of light sticks, a bag of #33 rubber bands, and a box of brass paper fasteners:

Method: Lay one end of a light stick across a rubber band: Pull both loops of the rubber band (sticking out to the right and to the left) up around the light stick.
Pull both loops of the rubber band (sticking out to the right and to the left) up around the light stick.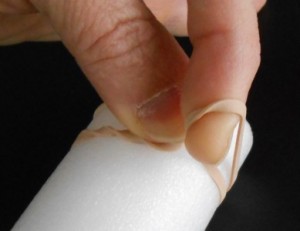 You want the rubber band to end up about 5 mm from the end of the stick. Pull one loop through the other,
You want the rubber band to end up about 5 mm from the end of the stick. Pull one loop through the other, and then tuck that loop once under the main part of the rubber band surrounding the light stick.
and then tuck that loop once under the main part of the rubber band surrounding the light stick. The rubber band should now stay in place due to friction with the foam, leaving a small loop sticking out at one spot, like this:
The rubber band should now stay in place due to friction with the foam, leaving a small loop sticking out at one spot, like this: Do that on both ends of a whole bunch of sticks.
Do that on both ends of a whole bunch of sticks.
To connect any number from two to five or even six sticks at a point, take each small rubber band loop sticking out, one from each stick, and slide it over the pointed tip of a paper fastener. When they are all on, bend the two “wings” of the paper fastener all the way back so they touch the flat head, trapping all of the rubber band loops. This provides a flexible but pretty sturdy link holding all the ends of the sticks together.
This provides a flexible but pretty sturdy link holding all the ends of the sticks together.
Recipes: 12 light sticks + 24 rubber bands + 6 paper fasteners = octahedron More interestingly, 26 light sticks plus 52 rubber bands plus 10 paper fasteners produces this model of the “Nechushtan spindle”, a graph that (notwithstanding the fact that it can be colored with five colors so that no two adjacent vertices are colored the same) plays a critical role in the ingenious proof that at least six colors are needed to color every point in space so that any two points which are a distance 1 apart are colored differently.
More interestingly, 26 light sticks plus 52 rubber bands plus 10 paper fasteners produces this model of the “Nechushtan spindle”, a graph that (notwithstanding the fact that it can be colored with five colors so that no two adjacent vertices are colored the same) plays a critical role in the ingenious proof that at least six colors are needed to color every point in space so that any two points which are a distance 1 apart are colored differently.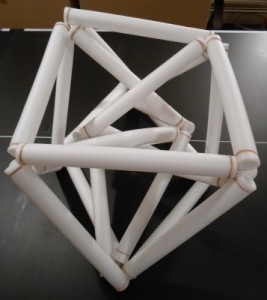 And here it is with the sticks doing their glowing light thing:
And here it is with the sticks doing their glowing light thing:

Other likely candidates for building in this way: the icosahedron, the snub cube, the rhombic dodecahedron, and the stellated rhombic dodecahedron. Of course, there are many other possibilities. As always, send a pic to mondays@momath.org to show us what you make!
This article first appeared on Make: Online, April 2, 2012.












