
________________
________________
Unleash Your Inner Rectangle
The midpoints of the sides of quadrilateral ABCD form the vertices of a rectangle. Angle CAB is 17 degrees.
What is the measure of angle ABD?
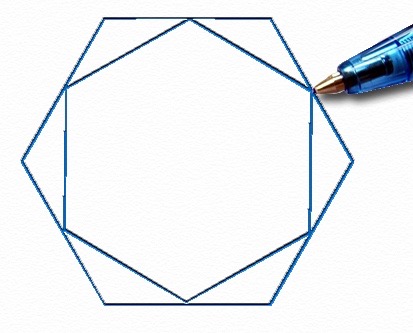
Pent-up-gon
The midpoints of the sides of pentagon ABCDE form the vertices of a regular pentagon.
What is the length of side BC divided by the length of side CD?
| Spread the word: | Tweet |
Solutions to week 75
Some Difference. First, let’s find the smallest and largest birthday sums to get an idea of how big the difference could possibly be. We’ll write dates like 68-2-18 for the example birthday, Feb 18, 1968, so that you can just add up the three numbers to get the birthday sum. The smallest possible birthday sum occurs for someone born on 00-1-1, namely 2; we find this by making each of the components as small as possible. Making each of the components as large as possible, the largest birthday sum, 142, goes to someone born on 99-12-31. But notice that 00-1-1 is the day after 99-12-31. So the largest possible difference of birthday sums of people born on consecutive days is 140.
In Your Prime. Note that after your first decade, all prime ages are odd, and 10k+5 is never prime (always divisible by five), so the only possibility for the k+1st decade to be prime is if all of 10k+1, 10k+3, 10k+7, and 10k+9 are prime. However, if k is a multiple of three, then 10k+3 is also a multiple of three, so that won’t be a prime decade. Similarly, if k is one less than a multiple of three, say 3j-1, then 10k+1 = 30j-10+1 = 30j-9 is a multiple of three. So only a decade for which k is one more than a multiple of three could work. We first try the forties, but 49 is not prime. Then we try the seventies, but 77 is not prime. The next possibility is the first decade after turning 100, and indeed, all of 101, 103, 107, and 109 are prime. Hence, your first prime decade after your teen years is your eleventh decade. Mathematically speaking, it’s clearly good to be a centenarian!
Recent Weeks
Week 75: Some Difference & In Your Prime, solutions to Frequent Figures & Doubled Digit
Week 74: Frequent Figures & Doubled Digit, solutions to Small Sudoku & Puny Pegs
Week 73: Small Sudoku & Puny Pegs, solutions to The Second Bisection & The Third Circle
Week 72: The Second Bisection & The Third Circle, solutions to Eye Like It & Pythagorean Poster
Week 71: Eye Like It & Pythagorean Poster, solutions to In The Groove & On The Path
Links to all of the puzzles and solutions are on the Complete Varsity Math page.
Come back next week for answers and more puzzles.
[asciimathsf]



