
________________
As a take-home treat, Coach Newton sends the team off for winter break with another set of problems inspired by the find of century-old geometry cards two weeks ago.
________________

Card 61
ACDF is a rectangle, with point B on side AC and E on side DF. Also lines BF and CE are parallel.
What fraction of the distance from A to C is point B if the area of quadrilateral BCEF is 1/7 of rectangle ACDF?
Card T
Points A and B lie on circle a with less than 90 degrees of arc between them. Circle b with center B goes through A and intersects a again at C. Circle c with center A goes through C and intersects b again at D.
How many times does line AD intersect circle a?
| Spread the word: | Tweet |
Solutions to week 66
Long Haul. An important technique in this problem is, rather than focusing on the lengths of each trip between mailboxes and adding those up, to consider the number of times each segment of the block between consecutive mailboxes can be traversed. The key is that another way of getting Piper’s total distance traveled is to add up for each such segment, how many times Piper walks it. For example, Piper can walk the first ten meters of the block (the part of the block from the first mailbox to the second) at most twice: to and from the first mailbox. Since Piper never detours when going between other mailboxes, there is nothing else that will make the path include that portion of the block. So that section of the sidewalk will contribute at most 20 total meters to Piper’s path.  Similarly, the last ten meters of the block can only be traversed twice, to and from the last mailbox, so that section also contributes at most 20 meters to the total path length. What about the second ten meters of the block, from the second mailbox to the third? Looking at the diagram on the left, you can see that this section can be traversed at most four times. In general if there at most k mailboxes to the left or to the right of a given stretch of sidewalk, Piper can only walk that section 2k times, going to and from each of those k mailboxes. Adding up each section of the sidewalk with the corresponding multiplier, Piper cannot walk more than 20+40+60+80+100+120+100+80+60+40+20 = 720 meters. However, it’s not actually possible to go quite that far, because all of those maximums assumed that Piper goes to and from every single mailbox, when in fact there is a starting mailbox that never has a trip to it and a final mailbox that never has a trip from it. In other words, we need to subtract at least the minimum separation of the starting and ending mailbox, which is ten meters (the distance from one mailbox to the next), to get an upper bound of 710 meters for the length of a trip. On the other hand, it turns out that it is possible to go this far: visiting the mailboxes in the order 7th-1st-8th-2nd-9th-3rd-10th-4th-11th-5th-12th-6th takes 60+70+60+70+60+70+60+70+60+70+60 = 710 meters, the maximum possible route length for Piper. (There are other routes that take the same total distance.)
Similarly, the last ten meters of the block can only be traversed twice, to and from the last mailbox, so that section also contributes at most 20 meters to the total path length. What about the second ten meters of the block, from the second mailbox to the third? Looking at the diagram on the left, you can see that this section can be traversed at most four times. In general if there at most k mailboxes to the left or to the right of a given stretch of sidewalk, Piper can only walk that section 2k times, going to and from each of those k mailboxes. Adding up each section of the sidewalk with the corresponding multiplier, Piper cannot walk more than 20+40+60+80+100+120+100+80+60+40+20 = 720 meters. However, it’s not actually possible to go quite that far, because all of those maximums assumed that Piper goes to and from every single mailbox, when in fact there is a starting mailbox that never has a trip to it and a final mailbox that never has a trip from it. In other words, we need to subtract at least the minimum separation of the starting and ending mailbox, which is ten meters (the distance from one mailbox to the next), to get an upper bound of 710 meters for the length of a trip. On the other hand, it turns out that it is possible to go this far: visiting the mailboxes in the order 7th-1st-8th-2nd-9th-3rd-10th-4th-11th-5th-12th-6th takes 60+70+60+70+60+70+60+70+60+70+60 = 710 meters, the maximum possible route length for Piper. (There are other routes that take the same total distance.)
Postal Pathways. You could drive yourself crazy trying to draw the diagrams of all of the different delivery routes.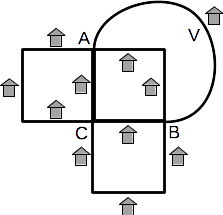 Instead, focus on the choices that Piper has to make in planning a route. To begin with, there are two directions to go from where the van is parked (at V in the diagram). Now, no matter which direction Piper goes from the van, the next choice will occur at a four-way intersection (either the one labeled A or the one labeled B). Since Piper can’t backtrack, there will be three choices for which way to go. When Piper gets to intersection C, there will similarly be three choices. And finally, whenever Piper first gets to the other intersection out of A and B, the one not immediately reached from the van, there will be only two choices for how to go, because if Piper headed to the van at the first opportunity, some houses would be left unvisited. On the other hand, when Piper returns to any of the intersections A, B, or C, there will only be one way left to go that has not yet been covered. So other than the choices listed, Piper’s path is completely determined. Multiplying the number of options at every choice point, there are at most 2×3×3×2 = 36 different routes Piper might take. We next have to verify which of these sequences of choices actually leads to a legal path that visits every house. It is easy to see that as long as Piper visits A and C before B, or B and C before A, then the route will never lead to a dead end, and all choices will be valid. On the other hand, if Piper visits both A and B before C (there are two ways to do this, A first from the van, then B, or B first from the van and then A), then not all three choices will work when Piper gets to C. For example, if Piper visits A then B then C and immediately goes back to B, the only route left at B is back to the van, leaving some houses unvisited. Conversely, if Piper visits B then A then C and immediately goes back to A, the same situation will occur at A. There are two direct routes from B to C (head either west from B or south from B), so there are two ways to visit A then B then C then B, and similarly, two ways two visit B then A then C then A. Hence four of the 36 sequences of choices that Piper might make do not lead to valid routes, and hence there are 32 valid routes that Piper can take.
Instead, focus on the choices that Piper has to make in planning a route. To begin with, there are two directions to go from where the van is parked (at V in the diagram). Now, no matter which direction Piper goes from the van, the next choice will occur at a four-way intersection (either the one labeled A or the one labeled B). Since Piper can’t backtrack, there will be three choices for which way to go. When Piper gets to intersection C, there will similarly be three choices. And finally, whenever Piper first gets to the other intersection out of A and B, the one not immediately reached from the van, there will be only two choices for how to go, because if Piper headed to the van at the first opportunity, some houses would be left unvisited. On the other hand, when Piper returns to any of the intersections A, B, or C, there will only be one way left to go that has not yet been covered. So other than the choices listed, Piper’s path is completely determined. Multiplying the number of options at every choice point, there are at most 2×3×3×2 = 36 different routes Piper might take. We next have to verify which of these sequences of choices actually leads to a legal path that visits every house. It is easy to see that as long as Piper visits A and C before B, or B and C before A, then the route will never lead to a dead end, and all choices will be valid. On the other hand, if Piper visits both A and B before C (there are two ways to do this, A first from the van, then B, or B first from the van and then A), then not all three choices will work when Piper gets to C. For example, if Piper visits A then B then C and immediately goes back to B, the only route left at B is back to the van, leaving some houses unvisited. Conversely, if Piper visits B then A then C and immediately goes back to A, the same situation will occur at A. There are two direct routes from B to C (head either west from B or south from B), so there are two ways to visit A then B then C then B, and similarly, two ways two visit B then A then C then A. Hence four of the 36 sequences of choices that Piper might make do not lead to valid routes, and hence there are 32 valid routes that Piper can take.
Thanks go to Varsity Math reader Geoffrey T. Fox for his correction concerning this problem; the solution originally erroneously stated that there were 36 possible paths for Piper.
Today’s pair of problems shows how, when you’re stuck on a question where you have to count something, try counting a different aspect of the problem than originally asked for: the number of times each section of sidewalk in Long Haul is crossed, or the number of choices in Postal Pathways.
Recent Weeks
Week 66: Long Haul & Postal Pathways, solutions to Card 129 & Card 94
Week 65: Card 129 & Card 94, solutions to Skimpy Schedule & Passing Pennies
Week 64: Skimpy Schedule & Passing Pennies, solutions to Seating Arrangement & Stuck on Fairness
Week 63: Seating Arrangement & Stuck on Fairness, solutions to Grid Adjustment & Way Outside the Box
Week 62: Grid Adjustment & Way Outside the Box, solutions to Further Fairness & Double-Crossed Training
Links to all of the puzzles and solutions are on the Complete Varsity Math page.
Come back next week for answers and more puzzles.
[asciimathsf]



