
________________
Piper, the neighborhood postal carrier, has lots of packages to deliver. The team tries to provide some diversion by turning Piper’s postal challenges into puzzles.
________________

Long Haul
One of the blocks where Piper delivers mail contains twelve houses in a row. All of the mailboxes are reachable from the sidewalk, and are equally spaced exactly ten meters apart. Piper visits each mailbox exactly once, possibly out of order, directly along the sidewalk from each mailbox visited to the next. The order in which she visits the houses determines the total distance Piper walks; for example, visiting the 2nd, 1st, 4th, 3rd, 6th, 5th, 8th, 7th, 10th, 9th, 12th, and 11th houses (in that order) requires walking 10 + 30 + 10 + 30 + 10 + 30 + 10 + 30 + 10 + 30 + 10 = 210 meters. On days when there’s a rush, Piper walks the least distance (110 meters) by visiting the houses in the usual order from first to twelfth. But what about when Piper wants the most exercise?
What is the greatest distance Piper can walk to deliver the mail on this block, measured from leaving the starting mailbox to reaching the last one?
Postal Pathways
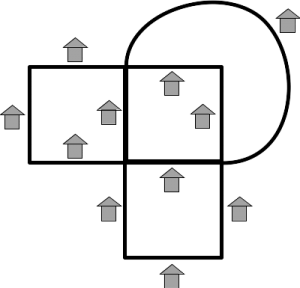 In another section of the neighborhood, the houses are very sparse; in fact, there’s just one on each block, as shown in the diagram. In this area, Piper always parks the postal van right in front of the house on the curved drive and delivers to each of the houses, staying on the roads, and returning to the van. Since the houses here are far apart, Piper always uses one of the shortest possible routes in which no section of road is traveled twice.
In another section of the neighborhood, the houses are very sparse; in fact, there’s just one on each block, as shown in the diagram. In this area, Piper always parks the postal van right in front of the house on the curved drive and delivers to each of the houses, staying on the roads, and returning to the van. Since the houses here are far apart, Piper always uses one of the shortest possible routes in which no section of road is traveled twice.
How many different possible routes are there that Piper can use to deliver the mail in this area?
| Spread the word: | Tweet |
Solutions to week 65
Card 129. The diagram at right shows the setup of the problem, with one point added, the midpoint M of segment BC. M is connected to D and E. With these auxiliary elements in place, it is easy to see that quadrilateral BCED is composed of three congruent copies of triangle EAD. Hence, the ratio of their areas is 3.
shows the setup of the problem, with one point added, the midpoint M of segment BC. M is connected to D and E. With these auxiliary elements in place, it is easy to see that quadrilateral BCED is composed of three congruent copies of triangle EAD. Hence, the ratio of their areas is 3.
Card 94. As you can see from the diagram below, a right triangle as described is just half of an equilateral triangle. Hence, angle ABC is 30°. It is a fact that any arc inscribed in an angle so that one end of the arc is tangent to one side of the angle at its vertex, as the shorter arc BD is in angle ABC, has measure twice the measure of the angle. So using that fact, we are done: the smaller arc BD therefore has measure 60°, so the longer arc BD has measure 360° – 60° = 300°. However, you can finish the puzzle even if you are not aware of this fact: drop the perpendicular from B to F on the other side of circle L. Then triangle BFD is a right triangle, being inscribed in a semicircle; and angle FBD is 60°, so angle BFD is 30°. But angle BFD is an inscribed angle cutting off the smaller arc BD of L. Hence, the measure of BD is 60° and the problem completes as before.

Both of today’s problems show the value of adding auxiliary information. So when you’re stuck on a problem, ask yourself, “What could I add to this diagram that might help?” or “What else can I figure out from the information given, even if it’s not exactly what the problem is looking for?”
Recent Weeks
Week 65: Card 129 & Card 94, solutions to Skimpy Schedule & Passing Pennies
Week 64: Skimpy Schedule & Passing Pennies, solutions to Seating Arrangement & Stuck on Fairness
Week 63: Seating Arrangement & Stuck on Fairness, solutions to Grid Adjustment & Way Outside the Box
Week 62: Grid Adjustment & Way Outside the Box, solutions to Further Fairness & Double-Crossed Training
Week 61: Further Fairness & Double-Crossed Training, solutions to Edible Division & Counting Cars
Links to all of the puzzles and solutions are on the Complete Varsity Math page.
Come back next week for answers and more puzzles.
[asciimathsf]



