________________
The Varsity Math team recently had a party to celebrate all of the summer birthdays of its members. Cameron missed the event, and when she asks about it she gets a couple of puzzles as responses.
________________
How Many
“How many team members attended the party?” is Cameron’s first question. A teammate responds, “Well, I cut the circular cake to make exactly one piece for each team member there. I used exactly nine straight vertical cuts that intersected the perimeter of the (top of the) cake at 18 distinct points. The cuts intersected in exactly seven places inside the circle, with just two cuts passing through each intersection.”
How many team members attended the party?
How Old
“Tell me more about the party,” says Cameron. “Well, among the birthdays we celebrated were those of one team member who graduated from high school early (but not more than two years early) and his two siblings not on the team.” Cameron is curious: “What are their ages?” The teammate tells Cameron the product of the three ages and that no first birthdays were celebrated, but Cameron complains that’s not enough information. “OK, well, the one oldest sibling still has not been old enough to vote in any general election.” “That eliminates some cases, but that’s still not enough information.” “Oh, and their actual birthdays happen to each be the fifth of the month, of June, July, and August, respectively.” Finally, Cameron is pleased to be able to figure out the ages of all three siblings.
How old is each of the siblings?
| Spread the word: | Tweet |
Solutions to week 46
Deck A Tile. There may be many shapes that possess the property indicated, but the wording of the problem strongly suggests that one can be found among the pentominoes, and that is in fact the case. Here are all of the pentominoes, and their flipped forms:
From left to right, the usual names for the pentominoes across the top are: I, F, L, P, and N. Those across the bottom are: T, U, V, W, X, Y, and Z. Let’s consider the possibilities for making rectangles from copies of each.
The I pentomino is already a rectangle by itself, so it cannot qualify. The F pentomino can never fill a rectangle. To see this, consider the upper-right-most pentomino in the rectangle. In order to fill the upper right square, it must be in the standard “F” orientation, as shown in the second illustration in the top row of the diagram. But now no copy of the F pentomino can fit in the 1×2 space left in the second and third squares of the rightmost column of the rectangle. Clearly 2 copies of the L pentomino make a recctangle, so it is not the one we’re looking for, and the same goes for the P. The N pentomino is another one that can never tile a rectangle. Again, imagine the upper-right-most pentomino. To fill the upper right corner, it must either be in the second-to-last orientation shown in the top row of the diagram, or rotated 180 degrees. Either way, it leaves a small “overhang” below it and to its left, that can only be filled by another N pentomino in a unique orientation. But these N pentominoes in turn each leave another overhang below and to the left, respectively, that must be filled by another N pentomino, leaving another overhhang, and so on. In other words, no matter how many N pentominoes you use, you can never “finish” the right or top edges of a rectangle; there is always an overhang left.
Proceeding to the second row, none of these except the Y pentomino can fill a rectangle. The upper-right-most T pentomino in a rectangle leaves a 1×2 gap in the rightmost column that cannot be filled, just as the F did. The empty square in the middle of a U pentomino can only be filled by another U rotated 180 degrees. Hence, if the U can fill a rectangle, so can a 3×4 rectangle missing two opposite corners. But the upper-right-most copy of this assembly leaves 1×1 gaps below and to its right that cannot be filled. 
The upper-right-most W leaves a 1×1 gap either below it or to its right that can only be filled by another W in a unique orientation. That leaves another 1×1 gap, which must be filled by another W leaving another 1×1 gap, and so on, so filling a rectangle is impossible. The X pentomino simply cannot fill the upper right square of any rectangle. And just like the F and the T, the Z pentomino leaves a 1×2 gap that cannot be filled. The most subtle non-rectangle-tiling pentomino is the V; see the diagram on the right. To fill the bottom right square of the rectangle, it must appear in the orientation and position labeled “A”. To fill in the square nested in the “elbow” of the V, i.e. the square one unit up and to the left of the bottom right, there must be another V in the orientation and position labeled “B”. This leaves overhangs to the left and above of A and B, which can only be filled by the V pentominoes labeled C and D. As a result, the elbow of B can only be filled by the pentomino labeled E, and now we are stuck: two 1×1 “holes” have been created in the elbows of C and D which cannot be filled.
That leaves only the Y pentomino. It can indeed form a rectangle, as shown in this diagram: 
As that uses 10 copies of the Y pentomino, we have established the solution, except we need to be sure that we cannot make a rectangle with any fewer copies of the polyomino. Since the pentomino has five squares, any rectangle will need to have one edge that is a multiple of five long. Color the squares along this edge red, orange, yellow, green, blue, in order, cycling among the five colors. Color the next row similarly, except starting with orange, and then cycling among yellow, green, blue, red, orange. Start each successive row with the next color in the cycle, and cycle through the colors in order. Since every row has a multiple of five squares, there are an equal number of each color. On the other hand, however you lay down a Y pentomino, it covers two of one color and is missing one of the colors. Hence, to end up with an equal number of each color, we must use a multiple of five Y pentominoes. Thus, the only smaller possible rectangle to be made with Y pentominoes is a 5-by-5 square. But such a square must have a long, flat side of the Y pentomino against each of its four sides, which does not leave room for a fifth Y pentomino. (The cavity will be one of the X, F, or Z pentominoes, not a Y.) Therefore, we conclude that Jules’ shape is the Y pentomino.
Try a Section.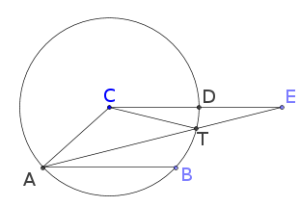 
See the diagram at left. Because triangle TCE is isosceles, angles TCE and TEC are equal. Moreover, both equal angle BAT because AB and CD are parallel. Since triangle CTA is isosceles, angle CAT equals angle CTA, which in turn is the sum of TCE and TEC since it is supplementary to angle ETC. Thus, angle CAT is exactly twice BAT, and hence BAT is one-third of CAB.
This was Archimedes’ solution to the problem of trisecting an angle with just compass and straightedge, except he had to cheat just a little bit and put a mark on his straightedge to get ET to be the same as AC. Thousands of years later, the angle trisection problem was proved impossible without some kind of cheat like Archimedes’.
Recent Weeks
Week 46: Deck a Tile & Try a Section, solutions to Boat Row & Meandering Mate
Week 45: Boat Row & Meandering Mate, solutions to Guarding Variety & As the Day is Wrong
Week 43: Relation Riddle & Crusty Conundrum, solution to The Old Coin Game
Week 42: Triangulating the Difference & The Old Coin Game, solution to A Day Late and A Fence Short
Links to all of the puzzles and solutions are on the Complete Varsity Math page.
Come back next week for answers and more puzzles.
[asciimathsf]
