
This week, some members of the Varsity Math team started out on an extended trip. Their first stop: the state fair.

Fractured Fruit
Two of the team members head toward a stand with a big sign proclaiming “Prize-winning peaches! Picked fresh today!” To their dismay, when they get there, the farmer is just taking down the sign. When they ask why, the response is “Sold out!” The teammates object, “But we’re only two hours into the fair.” The farmer responds, “Well, I sold four fifths of my peaches, plus two fifths of a peach, in the first half hour of the fair. And as it happens I sold four fifths of what remained plus two fifths of a peach in the second half hour of the fair. And remarkably, in the third half hour of the fair I sold four-fifths of what I had at the beginning of that half hour, plus two-fifths of a peach. And just in the past half hour I’ve sold four fifths of what was left after the first 90 minutes, plus two-fifths of a peach. And that resulted in all of my peaches being gone.” “That must have been messy, cutting up all of those peaches,” say the teammates. “Nope,” says the farmer, “I didn’t have to cut a single peach all morning.”
How many peaches did the farmer bring to the fair?
A Day Late and A Fence Short
Wandering away from the disappointing peach stand, the team members encounter a puzzled-looking fair official.  “What seems to be the problem?” they ask.”Well, you see, kids, we were supposed to have thirteen identical 10-foot fence panels to make six identical pens for the six finalists in the state hog-raising competition, as shown in this diagram. But somehow only twelve panels got on the truck. Now we’re not going to have anywhere to put the sixth pig.” The team members respond, “Don’t worry, we can show you how to rearrange things to make six identical pens with only twelve fence sections.” The official asks, “Are you sure? The owners say that each pig must have at least 40 square feet of space in its pen.” The team members assure the official that their plan will work.
“What seems to be the problem?” they ask.”Well, you see, kids, we were supposed to have thirteen identical 10-foot fence panels to make six identical pens for the six finalists in the state hog-raising competition, as shown in this diagram. But somehow only twelve panels got on the truck. Now we’re not going to have anywhere to put the sixth pig.” The team members respond, “Don’t worry, we can show you how to rearrange things to make six identical pens with only twelve fence sections.” The official asks, “Are you sure? The owners say that each pig must have at least 40 square feet of space in its pen.” The team members assure the official that their plan will work.
Draw the arrangement of fences that the team members suggest.


Solution to previous weeks
Duplicate Division. Since the order of the piles doesn’t matter, the team members are just counting the number of ways to express the number of trophies as the sum of four positive numbers, or once the Coach is included, as the sum of five positive numbers, and they get the same total either way. If we let `p(n,k)` be the number of ways to express `n` as the sum of `k` positive numbers, we are looking for `n` such that `p(n,4) = p(n,5)`. We can get an expression for `p(n,k)` as follows: either one of the numbers used is equal to one, or every number used is at least two. In the first case, there are `p(n-1,k-1)` possibilities: if you take one copy of “1” out of the sum, you get a way of expressing `n-1` as the sum of `k-1` numbers, and vice versa. In the second case, there are `p(n-k,k)` possibilities: you can subtract one from each part (since they are all at least two) to express `n-k` as a sum of `k` positive numbers. In other words, `p(n,k) = p(n-1,k-1) + p(n-k,k)`. A few other facts are handy: `p(n,1) = 1` for any `n`, since to express `n` as a sum of one number, you must simply use `n` itself; `p(n,n) = 1`, since to express `n` as a sum of `n` positive numbers, they must all be one; and `p(n,m) = 0` when `m > n`, since there is no way to express `n` as the sum of more than `n` positive whole numbers. These relations let us quickly fill out a table of `p(n,1)` through `p(n,5)` for various `n`, since they never refer to values of `p` in which `k` is larger than 5. We get
| n |
p(n,1) |
p(n,2) |
p(n,3) |
p(n,4) |
p(n,5) |
| 1 |
1 |
0 |
0 |
0 |
0 |
| 2 |
1 |
1 |
0 |
0 |
0 |
| 3 |
1 |
1 |
1 |
0 |
0 |
| 4 |
1 |
2 |
1 |
1 |
0 |
| 5 |
1 |
2 |
2 |
1 |
1 |
| 6 |
1 |
3 |
3 |
2 |
1 |
| 7 |
1 |
3 |
4 |
3 |
2 |
| 8 |
1 |
4 |
5 |
5 |
3 |
| 9 |
1 |
4 |
7 |
6 |
5 |
| 10 |
1 |
5 |
8 |
9 |
7 |
| 11 |
1 |
5 |
10 |
11 |
10 |
| 12 |
1 |
6 |
12 |
15 |
13 |
| 13 |
1 |
6 |
14 |
18 |
18 |
| 14 |
1 |
7 |
16 |
23 |
23 |
| 15 |
1 |
7 |
19 |
27 |
30 |
| 16 |
1 |
8 |
21 |
34 |
37 |
| 17 |
1 |
8 |
24 |
39 |
47 |
| 18 |
1 |
9 |
27 |
47 |
57 |
| 19 |
1 |
9 |
30 |
54 |
70 |
So evidently there are two possibilities for the number of trophies: 13 or 14 and no way to distinguish between the two, so we conclude that Duplicate Division has duplicate answers.
Coach Newton weighs in:
I hope you’ve noticed we’re not quite done, are we? We found two possible values for the number of trophies, how do we know there aren’t any more? It certainly appears from the table that `p(n,5)` is growing faster than `p(n,4)`, and that’s exactly what we will show: for `n >= 19`, `p(n,5) – p(n-1,5) > p(n,4) – p(n-1,4)`. That will guarantee they could never be equal again.
To see that fact, it will help to have a word to mean “a way of expressing `n` as a sum of positive integers” — mathematicians call that a “partition of `n`”, and each of the positive integers used is called a “part” of the partition. So first notice that if you take any partition of `n-1` into four parts, and you add one to the largest part, you get a partition of `n` into four parts, with the property that the two largest parts are guaranteed to be different. Moreover, if two partitions of `n-1` are different, and you do this same thing to both of them, you get different partitions of `n`; and every partition of `n` with the two largest parts different comes up this way, since it comes from the partition of `n-1` produced by subtracting one from its largest part. This shows that `p(n,4) – p(n-1,4)` is precisely the number of partitions of n into 4 parts with the two largest parts the same. And there was nothing special about four; we have the exact same property with five in place of four. So what we will show is that for `n >= 19`, there are at least as many partitions of `n` into five parts with the two largest parts the same as there are into four parts with the two largest parts the same.
Now we do something called taking the “conjugates” of those partitions, which I will let you look up on the web, which tells you that the number of partitions of `n` into four parts, the two largest parts the same, is the same as the number of partitions of `n` into parts all at least two, the largest of which is four. And similarly for five parts, we get the number of partitions of `n` into parts all at least two, the largest of which is five. We will show the latter number is no smaller, and we will do that by mapping each of the partitions with largest part four into one with largest part five so that no two of the former get mapped into the same partition, so there have to be at least as many of the latter type.
Here’s how you do it: If the starting partition (each part at least two, largest part four) has a three in it, change that three to a two and add one to the four, to get a partition with exactly one five in it. If the starting partition has no three, but has at least three fours in it, change one of the fours to a two and add one to each of two other fours, to get a partition with exactly two fives in it. We know we won’t get any collisions so far, because we can work backward: given a partition with exactly one five in it, we subtract one from the five and add it on to the smallest part to get the partition it must have come from, and if it has exactly two fives in it, we subtract one from each and add two to a two in the partition to produce a four. But we haven’t quite exhausted all of the partitions with each part at least two, largest part four, yet — there could be partitions with no three, and that do not have at least three fours. But there are at most two of these: one with one four, and all the rest twos, and the other with two fours, and all the rest twos. But since `n>=19`, there will be at least two partitions of `n` that have exactly three fives and each part at least two, and we have not used those yet, so we can map the remaining partitions with largest part four into those.
Hence, there are at least as many partitions of ‘n’ with largest part five as with four, all parts at least two. And by the chain of reasoning above, that means there can’t be any more solutions to Duplicate Division.
Stamp Stumper. To find the largest amount of postage, just start with the largest denomination of stamp you’re allowed to use and try to have the most of that denomination that you can, and work your way down. So if we use the answer 13 from Duplicate Division, we start with 13-cent stamps, and we can have at most 12 of them, since the number must be the denomination of a different kind of stamp. Turning next to the 12-cent stamps, we want to maximize those, so we would use 13 of those stamps. We proceed in the same manner: there can be at most 10 11-cent stamps, and 11 10-cent stamps; and then at most 8 9-cent stamps, and 9 8-cent stamps. This gives a total of 13×12 + 12×13 + 11×10 + 10×11 + 9×8 + 8×9 = 676 cents.
If you use the answer 14 from Duplicate Division, things go almost the same way. You start with 13 14-cent stamps, then 14 13-cent, 11 12-cent, 12 11-cent stamps, and 9 10-cent stamps. But if we used 10 9-cent stamps, we would not be able to use any 8-cent stamps (because the number of 8-cent stamps has to be the denomination of a different kind of stamp, and all of the others would be used up). So instead it is better to use 8 9-cent stamps and 10 8-cent stamps, for a total of 14×13 + 13×14 + 12×11 + 11×12 + 10×9 + 9×8 + 8×10 = 870 cents.
Awkward Positions. To begin with, the number of team members is supposed to be the square root of the answer to Stamp Stumper, and now only the 676 works, since 870 is not the square of a whole number. So we conclude that there are 26 (=√676) team members, and we want the probability that if they are sitting around a table and we choose four at random, that two are sitting next to each other. However, it’s easier if we imagine that the 22 that are not picked are sitting around the table, what’s the probability that if we insert four at random, that two end up sitting next to each other. And it’s even easier if we now calculate the probability that no two end up next to each other, and then subtract that from 1. To calculate this, please note that with 22 people sitting around the table, there are 22 spaces between people where the next person could sit, then 23 for the person after that, 24 for the person after that, and 25 for the last person to sit. In other words, there are 22×23×24×25 ways to insert four additional people around the table, in all. In how many of these ways do no two of them end up sitting next to each other? Well, the first person can sit anywhere, so there are still 22 spots; but now the spots immediately to the left and right of that are disqualified, so there are only 21 spots the next person can sit in, which creates one more spot but disqualifies two of them, for 20 spots for the third person and finally 19 possibilities for where the last person could sit. Therefore, the probability (if the people are seated at random) that no two end up next to each other is 22×21×20×19 / 22×23×24×25 = 133/230. And the requested probability is one minus this number, or 97/230.
Sine of Success. 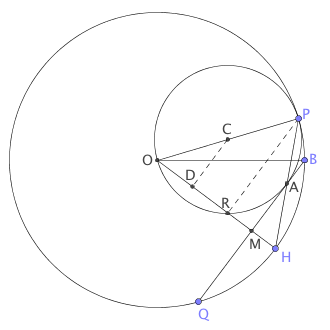 We replace the players by points with the same initials `B, Q, P,` and `H` on a circle of radius five. The distance `BQ` is 6, and `H` is the only point such that chord `PH` is bisected by `BQ`. So consider the locus of the midpoints of all of the chords from `P` to some other point on the circle. This locus is a smaller circle with diameter `PO`, where `O` is the center of the original radius-five circle. And since there is only one point `H` where `PH` is bisected by `BQ`, we conclude that `BQ` must touch this smaller circle exactly once — i.e., `BQ` is tangent to the smaller circle. All these observations lead to the diagram at right, in which some additional important points have been labeled, in particular `C`, the center of the smaller circle; `M`, the midpoint of `BQ`, and `D` and `R`, the perpendicular projections of `C` and `P` onto `OM`. We want the sine of angle `BOP`, and our approach will be to find the sines and cosines of `/_POM` and `/_BOM` and use the angle difference formula for sine. To that end, we attempt to write down the length of as many sides of the two right triangles `POM` and `BOM` as possible: it is given that `PO` and `BO` are 5 and that `BM` is 3, hence by the Pythagorean theorem, `OM` is 4. Now, `CAMD` is a rectangle, so `MD` = `AC` = 5/2, so `OD = OM – MD` = 3/2. By similar triangles, since `PO` is twice `CO`, `OR` is 3, and hence `PR` is 4 (another 3-4-5 triangle!). Finally, using the difference formula, `sin POB = sin POM cos BOM – sin BOM cos POM` = (4/5)(4/5) – (3/5)(3/5) = 7/25.
We replace the players by points with the same initials `B, Q, P,` and `H` on a circle of radius five. The distance `BQ` is 6, and `H` is the only point such that chord `PH` is bisected by `BQ`. So consider the locus of the midpoints of all of the chords from `P` to some other point on the circle. This locus is a smaller circle with diameter `PO`, where `O` is the center of the original radius-five circle. And since there is only one point `H` where `PH` is bisected by `BQ`, we conclude that `BQ` must touch this smaller circle exactly once — i.e., `BQ` is tangent to the smaller circle. All these observations lead to the diagram at right, in which some additional important points have been labeled, in particular `C`, the center of the smaller circle; `M`, the midpoint of `BQ`, and `D` and `R`, the perpendicular projections of `C` and `P` onto `OM`. We want the sine of angle `BOP`, and our approach will be to find the sines and cosines of `/_POM` and `/_BOM` and use the angle difference formula for sine. To that end, we attempt to write down the length of as many sides of the two right triangles `POM` and `BOM` as possible: it is given that `PO` and `BO` are 5 and that `BM` is 3, hence by the Pythagorean theorem, `OM` is 4. Now, `CAMD` is a rectangle, so `MD` = `AC` = 5/2, so `OD = OM – MD` = 3/2. By similar triangles, since `PO` is twice `CO`, `OR` is 3, and hence `PR` is 4 (another 3-4-5 triangle!). Finally, using the difference formula, `sin POB = sin POM cos BOM – sin BOM cos POM` = (4/5)(4/5) – (3/5)(3/5) = 7/25.
Recent Weeks
Week 40: Awkward Positions & Sine of Success, solution to Spiral Notebook
Week 39: Stamp Stumper & Spiral Notebook, solution to Phenomenal Packing
Week 38: Phenomenal Packing & Duplicate Division, solutions to Hat Trick, Possible Parabolas, Equal Laterals, & Isocircules
Week 37: Equal Laterals & Isocircules, solution to Circle Crossing
Week 36: Possible Parabolas & Circle Crossing, solution to Heart Trick
Week 35: Hat Trick & Heart Trick, solutions to Test Test, Simultaneous Remainders, Different Dice, & No-Dispute Knights
Week 34: Different Dice & No-Dispute Knights, solution to Subtle Sequences
Links to all of the puzzles and solutions are on the Complete Varsity Math page.
Come back next week for answers and more puzzles.
[asciimathsf]
 “What seems to be the problem?” they ask.”Well, you see, kids, we were supposed to have thirteen identical 10-foot fence panels to make six identical pens for the six finalists in the state hog-raising competition, as shown in this diagram. But somehow only twelve panels got on the truck. Now we’re not going to have anywhere to put the sixth pig.” The team members respond, “Don’t worry, we can show you how to rearrange things to make six identical pens with only twelve fence sections.” The official asks, “Are you sure? The owners say that each pig must have at least 40 square feet of space in its pen.” The team members assure the official that their plan will work.
“What seems to be the problem?” they ask.”Well, you see, kids, we were supposed to have thirteen identical 10-foot fence panels to make six identical pens for the six finalists in the state hog-raising competition, as shown in this diagram. But somehow only twelve panels got on the truck. Now we’re not going to have anywhere to put the sixth pig.” The team members respond, “Don’t worry, we can show you how to rearrange things to make six identical pens with only twelve fence sections.” The official asks, “Are you sure? The owners say that each pig must have at least 40 square feet of space in its pen.” The team members assure the official that their plan will work.





