
________________
________________

Nine Coins
The coach provides the team with nine coins that are identical in appearance, telling them that three of the coins weigh 3 units each, three weigh 2 units each, and three weigh 1 unit each. Hannah knows what each coin weighs, but needs to convince the coach that she knows.
How many weighings on a simple two-pan balance does she need to make to convince the coach that she knows which coins are which?
Five Questionable Coins
Ethan is holding a true coin and is told that among five identical looking coins on the table, one is counterfeit. The counterfeit is heavier or lighter than a true coin.
How can he find the counterfeit in two weighings using a simple two-pan balance?
| Spread the word: | Tweet |
Solutions to week 107
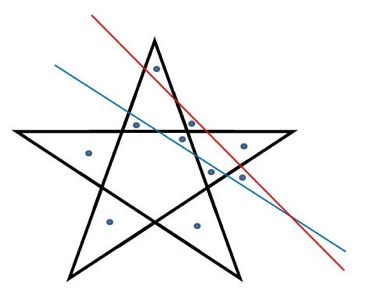
The answer for Ten from Two involves thinking outside the box (or more properly, thinking outside the star; see below). The answer for Triangular Boundary is 18.
Ten from Two answer explained:
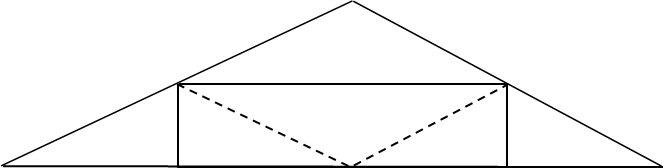
Triangular Boundary answer explained: For any triangle with sides a ≤ b ≤ c, the maximum area of the inscribed rectangle is equal to half the area of the triangle. As shown below, place the corners of the rectangle at the midpoints of the two shorter sides and the rectangle’s opposite side flush with the longest side of the triangle. Consider the triangle as a piece of paper and imagine folding it over the edges of the rectangle, as indicated by the dashed lines. Note that the folds will exactly cover the rectangle of maximum area. For any other rectangle within the triangle, folding over the uncovered pieces of the triangle will produce full coverage of the rectangle plus some overlap, overhang, or both, showing that the rectangle is not of maximum area.
The area of any triangle with sides a, b, and c is √ [s(s – a)(s – b)(s – c)], known as Heron’s formula, where s is one half of the perimeter. For sides of 9, 10, and 17, s = 18, s – a = 9, s – b = 8, and s – c = 1. The area of the triangle is therefore √ [18*9*8*1], or 36, and the area of the maximum rectangle is half the area of the triangle, or 18.
Recent Weeks
Week 107: Ten from Two & Triangular Boundary, solutions to Simple Multiple & Small and Simple
Week 106: Simple Multiple & Small and Simple, solutions to Math for the Ages & Three Racers
Week 105: Math for the Ages & Three Racers, solutions to X Factor & Perfect Pairings
Week 104: X Factor & Perfect Pairings, solutions to Two Sums & Double-Faced
Week 103: Two Sums & Double-Faced, solutions to Zombie Epidemic & Revenge on the Zombies
Links to all of the puzzles and solutions are on the Complete Varsity Math page.
Come back next week for answers and more puzzles.














