
________________
With the election nearing, the Varsity Math team decides to explore some puzzles that relate to voting of various kinds.
________________

Nearly Invincible
A company with 97 shares has five shareholders. (Each shareholder owns a whole number of shares, and the holdings of all of the shareholders add up to exactly 97.) A winning coalition is a subset of the shareholders who together own more than half of the shares. One particular shareholder is a member of every winning coalition except for precisely one. As it happens, that shareholder owns the minimum number of shares that makes it possible to be a member of every winning coalition but one.
How many shares does that shareholder own?
Extremely Equal
Some members of the team get together and decide to vote on which topics are best and worst for math problems, with just three available options. (Assume that each team member has a personal ranking of the three topics, without ties, and honestly votes his or her top-ranked one as “best” and bottom-ranked one as “worst”.) They count all the ballots for “best” and find that one topic has more votes than either of the others. The teammates think it’s great that they’ll be able to announce the best topic to the coaches, until they go through again and discover that more than half of the ballots list that same topic as worst! In classic Varsity Math style, one of the attendees observes, “Too bad nobody else showed up. With one more person voting, this annoying coincidence couldn’t have happened, even if we changed our votes.”
How many “worst” votes did the winning topic receive?
| Spread the word: | Tweet |
Solutions to week 57
Three Circles, One Distance. 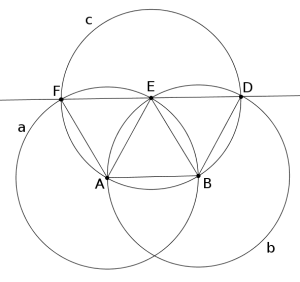 Because circles a and b have the same radius, triangle EAB is an equilateral triangle regardless of the choice of A and B, but if we take those two points to be 60° apart, then segment AB becomes, as shown to the right, one side of an inscribed regular hexagon, E becomes the center of the circle, triangles EAF and BED become equilateral also, DF becomes a diameter of the circle, and it is clear that DE has length 3, the radius of circle c.
Because circles a and b have the same radius, triangle EAB is an equilateral triangle regardless of the choice of A and B, but if we take those two points to be 60° apart, then segment AB becomes, as shown to the right, one side of an inscribed regular hexagon, E becomes the center of the circle, triangles EAF and BED become equilateral also, DF becomes a diameter of the circle, and it is clear that DE has length 3, the radius of circle c.
On the other hand, even though we don’t need to for finding the answer, Coach Newton might like it if we checked up on the folks writing the problem and figured out why length DE won’t change as we vary points A and B. 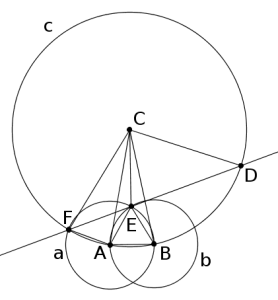 For that, refer to the diagram at left, where A and B are chosen at a different separation, E is no longer at the center of the circle, and so we’ve added point C to be the center of c. We’d like to show that triangle DCE is isosceles, and so the plan will be to show that angles DCE and DEC are equal.
For that, refer to the diagram at left, where A and B are chosen at a different separation, E is no longer at the center of the circle, and so we’ve added point C to be the center of c. We’d like to show that triangle DCE is isosceles, and so the plan will be to show that angles DCE and DEC are equal.
To do this, let the measure of congruent angles EAC and EBC be x. Since line EC is the perpendicular bisector of AB, angles CEA and CEB are congruent and hence both equal to 150°. Therefore angles ECA and ECB are both 30-x degrees. Angles CFA and CAF are congruent to CAB and so have measure 60+x degrees. Therefore, angle EAF is 60+2x degrees. Also, triangle EAF is isosceles, so angle EFA is 60-x degrees. Subtracting that from CFA gives us that angle CFE is 2x. Since angle FCA is congruent to ACB, FCE is three times ACE, or 90-3x. That leaves angle CEF as 90+x, so angle DEC is 90-x.
It remains to chase down angle DCE. But triangle DCF is isosceles, and we know angle CFE is 2x. Hence angle DCF is 180-4x. Subtracting angle FCE, we get that angle DCE is also 90-x degrees. Hence triangle DCE is isosceles, and DE is equal to the radius of circle c, or 3, as desired.
Great! And there’s even more. This arrangement of circles gives you a cool way to find the center of any circle, which will play the role of c in this problem. Pick any two points A and B on the given circle, draw circles a and b and line DEF, and also draw the line between both points of intersection of a and b. That’s the perpendicular bisector of chord AB, and so contains the desired center C. But from the above we know that the circle with center D that goes through E also goes through C, so draw that circle, and where it intersects the perpendicular bisector must be the center of the circle. This is a practical method to find the center of a circular piece of paper, for example, if you’ve got a compass and ruler.
Circle Stack.  Although one cylinder could go into the box diagonally, it would then interfere with the other cylinder and make it rise higher. Therefore, we can best pack the cylinders into the box with their long axes parallel to the long sides of the box. If we now look at a cross section of the box perpendicular to that long axis, it looks like the accompanying diagram. Here, A is the center of the 17-cm radius pipe, B is the center of the 9-cm radius pipe, and C is the point directly to the right of A and directly below B. The dotted line represents the lid of the shallowest box into which the cylinders will fit. Segment AC is 24 cm, because it is the width of the box (50 cm) minus the two radii (17 cm and 9 cm). And segment AB is the sum of the two radii, or 26 cm. By construction, triangle ABC is a right triangle, so we can use the Pythagorean theorem to deduce that segment CB is √(26² – 24²) = 10 cm long. And hence, the box must be 17 cm + 10 cm + 9 cm = 36 cm deep.
Although one cylinder could go into the box diagonally, it would then interfere with the other cylinder and make it rise higher. Therefore, we can best pack the cylinders into the box with their long axes parallel to the long sides of the box. If we now look at a cross section of the box perpendicular to that long axis, it looks like the accompanying diagram. Here, A is the center of the 17-cm radius pipe, B is the center of the 9-cm radius pipe, and C is the point directly to the right of A and directly below B. The dotted line represents the lid of the shallowest box into which the cylinders will fit. Segment AC is 24 cm, because it is the width of the box (50 cm) minus the two radii (17 cm and 9 cm). And segment AB is the sum of the two radii, or 26 cm. By construction, triangle ABC is a right triangle, so we can use the Pythagorean theorem to deduce that segment CB is √(26² – 24²) = 10 cm long. And hence, the box must be 17 cm + 10 cm + 9 cm = 36 cm deep.
Recent Weeks
Week 57: Three Circles, One Distance & Circle Stack, solutions to Equitable Area & Angular Equity
Week 56: Equitable Area & Angular Equity, solutions to Diagonalization & Regionalization
Week 55: Diagonalization & Regionalization, solutions to Watch for Falling Nuts & Fall Out of Line
Week 54: Watch for Falling Nuts & Fall Out of Line, solutions to Hexadiagonal & Hexintersection
Week 53: Hexadiagonal & Hexintersection, solutions to Mean Triangle & As Easy as 4132
Links to all of the puzzles and solutions are on the Complete Varsity Math page.
Come back next week for answers and more puzzles.
[asciimathsf]



