
________________
Most of the Varsity Math team is back in the town Logiton, where the authorities need their help again.
________________
Guarding Variety
Guarding Variety
First, a private security firm asks them for assistance. 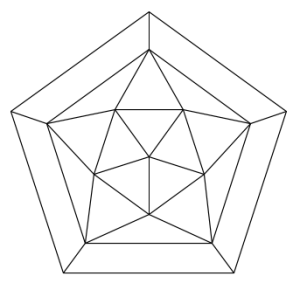 “You guys, old Julius Petersen, owner of Pentagon Mansion on the outskirts of town, is having his annual open house. He’s hired us to guard his eclectic collection of objets d’art. In his crazy floor plan [right]; there are doors in every internal wall. If anybody swipes anything, a silent alarm will immediately lock down the only door out of the building, but then we have to catch the thief. My guards might be anywhere when the theft happens, and it could happen in any room. After that, first the thief and then each of the guards will be able to move from any room to any adjacent room (or stay put) once each minute. We can track the thief on video surveillance, but we need to get a guard into the same room as the thief at some point to apprehend him. I have to pay my guards a lot for an assignment like this, so I want to keep the staffing down.”
“You guys, old Julius Petersen, owner of Pentagon Mansion on the outskirts of town, is having his annual open house. He’s hired us to guard his eclectic collection of objets d’art. In his crazy floor plan [right]; there are doors in every internal wall. If anybody swipes anything, a silent alarm will immediately lock down the only door out of the building, but then we have to catch the thief. My guards might be anywhere when the theft happens, and it could happen in any room. After that, first the thief and then each of the guards will be able to move from any room to any adjacent room (or stay put) once each minute. We can track the thief on video surveillance, but we need to get a guard into the same room as the thief at some point to apprehend him. I have to pay my guards a lot for an assignment like this, so I want to keep the staffing down.”
What is the smallest number of guards that will guarantee that any single thief can be apprehended within a finite number of minutes?
As the Day is Wrong
Next, the police chief needs the team’s help. He’s rounded up two members of Non Sequitur, the crime ring in Logiton, because he’s learned the group is planning to steal the platinum doorknobs of Pentagon Mansion next week. Here’s the interrogation of the two ring members, known only as X and Y.

Chief: So when is this job going down?
Y: Saturday.
X: No, Sunday.
Chief: Hmmm. You’re going to need to fence the doorknobs the day after the crime. What day will that be?
X: Tuesday.
Y: No, Wednesday.
Chief: This isn’t making sense! OK, you’ll need to case the Mansion the day before the crime. When will that be?
Y: Thursday.
X: No, Friday!
Then the Chief gets a big break: He learns that the peculiar code of honor that X and Y follow requires each of them to give exactly one true answer out of every three questions they’re asked.
Which day will Non Sequitur attempt to rob Pentagon Mansion?
| Spread the word: | Tweet |
Solution to week 43
Fractured Fruit. I’m sure Coach Newton would suggest working backwards on a problem like this, where you end up in a known state (all peaches gone) after a series of operations. To end up with no peaches left after the fourth half hour, in which four fifths of the peaches were sold plus two fifths of a peach, that two fifths of a peach must exactly match what was left from four fifths of the peaches. In other words, the other one fifth of the peaches must be that two fifths of a peach. For that to be the case, there must have been exactly two peaches remaining at the start of the fourth half hour. Continuing to work backwards, let x be the number of peaches at the beginning of the third half hour, and use the same logic as before. We know that that number of peaches left at the end of the third half hour is (1/5)x – 2/5, which must match the 2 peaches left at the beginning of the fourth half hour. As an equation, (1/5)x – 2/5 = 2. To solve this, we multiply by five and add two, to get x = 5×2 + 2 = 12.
To continue this process, notice that the 2 peaches left at the end of the third half hour could have been any number — to get the amount there were at the beginning of the hour, you can multiply by five and add two. So at the beginning of the second half hour there were 5×12 + 2 = 62 peaches, and at the very beginning of the day there were 5×62 + 2 = 312 peaches.
Triangulating the Difference. Based on the answer to the previous puzzle, we have fifteen cards labeled 1 through 15 and must lay them down in a triangle such that the number on each card is the difference of the two numbers resting on it. How can we start? Well, 15 is not the difference of any two smaller numbers, so 15 must occur in the top row (where it does not have to be the difference of two numbers). Also, note that the difference of an odd and an even is odd, while the difference of two evens or two odds is even. But there are 8 odd numbers and 7 even numbers from 1 to 15, so by trying the 20 odd/even patterns for the top row (up to reflection in the vertical axis of the triangle), it is not hard to see that the top row must have one of these five patterns: OEOEE, OEEEO, OOOEE, OOEEO, or OOOOE.
Now, imagine you had the filled in triangle satisfying the rules. The bottommost number is the difference of the two above it. The larger (L) of the two above is the sum of the number next to it and the bottommost. Consider the two numbers above L. The larger of those two numbers is the sum of the nunber next to it and L. Continue working your way up the rows, always looking at the larger number above the one from the previous row. It is always the sum of its adjacent number and the one below it. Chaining all of these sums together, there is a number in the top row which is the sum of one number from each of the rows. But the only number less than or equal to 15 which is the sum of five different numbers less than or equal to 15 is 15 = 1+2+3+4+5. So we conclude that one each of 1 through 5 lie in each of the five rows. Moreover, since those are the smallest numbers in the triangle, each one lies next to the biggest number in its row.
Now we can start to analyze the locations of the next bigger numbers. 14 is either in the top row, or it comes from 15 adjacent to 1. If it is below 15 and 1, then the 13 must be in the top row, because it can’t come from 14 and 1 (the 1 is used) and it can’t come from 15 and 2 (because 1 and 2 must be in different rows). If 15 and 1 are adjacent in the top row and 13 is also in the top row, then there are three possibilities. Possibility A: the 13 is next to the 1, so that the top row has 15 1 13 in it, the next has 14 12, and the 2 is in the third row. Then the only place the 11 can go is next to the 15, giving 11 15 1 13 (E) for the top row, leading to 4 14 12 (O), 10 2 (O), and 8 (O) for the following rows, where (E) and (O) represent even and odd numbers respectively. But then the only even number left for the top row is 6, and putting that on either side of 11 15 1 13 in the top row leads to some number being repeated. Possibility B: the 13 is next to the 15. Again, the 11 will have to also be on the top row, yielding 13 15 1 11 (E) on top. Then the following rows are 2 14 10 (O), 12 4 (O), and 8 (O). Again, the only even number left is 6, and adding that at either side of 13 15 1 11 causes a repeat somewhere in the triangle. Possibility C: the 13 is not adjacent to either the 15 or the 1. But then the 12 will have to end up in the top row (because there is no way it can arise from the 13, 14, or 15 in this case.) Further, the 12 cannot be adjacent to the 13, since that would produce a duplicate 1. With the possible odd/even patterns, that leaves only 15 1 12 (E) 13 for the top row, and now there is no way to generate a 10 from this top row, nor does 10 work as the remaining even number in the top row (it would yield a duplicate 1 in the third row).
Since none of possibilities A, B, and C hold, we conclude that 14 is not in the second row, so it must be in the top row.
OK, so can 15 be adjacent to 2 in the top row, putting 13 in the second row and 14 elsewhere in the top row? If so, then where could the 12 be? There are two options. Possibility D: the 14 is adjacent to the 2, so that part of the triangle has 15 2 14 above 13 12 above 1. Then 11 must also go on the top row (since it can’t come from any of 12 through 15 in this configuration), and the only way to get the 10 is to have a 3 next to the 13. But that would involve repeating the 12 next to the 15 to produce that 3, so D turns out to be impossible. Possibility E: The 14 is not adjacent to the 2, and the 12 occurs elsewhere on the top row. But there are only two patterns for the top row with three even positions, so we would have to have either 15 2 (O) 12 14, which would duplicate the 2 in the second row, or (O) 2 15 12 14, which has the same problem, or (O) 14 15 2 12, which leads to a duplicate 12 in the third row, or 15 2 12 14 (O), which duplicates the 2 in the second row. Since neither possibility D or E works, we conclude that 15 is not adjacent to 2 in the top row, so the 13 cannot be in the second row, so it must also be in the top row along with 15 and 14.
Finally, can 15 be adjacent to 3 in the top row, putting 12 in the second row? The top row would have 15, 14, 13, and 3 in this case, which is almost all of its entries. Given the few patterns of odds and evens, there are now only a few possibilities to check to discover that the magician’s layout must have been
| 13 | 3 | 15 | 14 | 6 | ||||
| 10 | 12 | 1 | 8 | |||||
| 2 | 11 | 7 | ||||||
| 9 | 4 | |||||||
| 5 |
(To fully show that this is the only possible solution, a bit more analysis to eliminate the possibilities of a 4 or 5 in the top row instead of the 3 must be performed.)
Crusty Conundrum. The ancient Greek geometer Archimedes discovered that the surface area of any portion of a sphere cut by two parallel planes is equal to the surface area of the section of the circumscribing cylinder of the sphere cut by those same two planes. Hence, the crust on each slice of the bread is simply proportional to its thickness (the distance between the two planes that define the slice). Since the radius of the slices furthest out to the end is smallest, those slices must be the thickest to have the same volume as the other pieces. Hence, either of the end slices will have the most crust (regardless of how many pieces the sphere is cut into; in other words, this problem can be solved without solving the prior one in the relay).
Relation Riddle. Note that the two grandparents and the three grandchildren must all be different people. Also, since none of the grandchildren can be parents (without introducing additional people), there must be an additional father and mother present. To avoid any additional guests, the two grandparents must be the parents of one of the parents of the grandchildren. To avoid introducing another person to be the son-in-law, we conclude that the two grandparents must be the parents of the grandchildren’s mother. And finally, to avoid introducing anyone new to be the brother-in-law, we conclude that Finley is the sibling of that mother, so that her husband is the brother-in-law. That means that the guests at the reunion that day were Finley’s parents, sister, sister’s husband, and two nieces and one nephew (children of the sister).
Recent Weeks
Week 43: Relation Riddle & Crusty Conundrum, solution to The Old Coin Game
Week 42: Triangulating the Difference & The Old Coin Game, solution to A Day Late and A Fence Short
Week 40: Awkward Positions & Sine of Success, solution to Spiral Notebook
Week 39: Stamp Stumper & Spiral Notebook, solution to Phenomenal Packing
Week 37: Equal Laterals & Isocircules, solution to Circle Crossing
Week 36: Possible Parabolas & Circle Crossing, solution to Heart Trick
Links to all of the puzzles and solutions are on the Complete Varsity Math page.
Come back next week for answers and more puzzles.
[asciimathsf]

