Patriotic Puzzles
In honor of the Fourth of July, MoMath presents some intriguing patriotic puzzles.
1. Which U.S. state is closest to Africa?
2. There are three pairs of balls — red, white, and blue. In each pair, one ball is a little bit heavier than the other one. All the heavy balls weigh the same, and all the light balls weigh the same. You have a simple balance scale that can distinguish only whether both pans hold the same weight or different weights. In just two weighings, you have to determine the light and the heavy balls in each pair. How can it be done?
3. What is the biggest city in the United States east of Reno, NV and west of Denver, CO?
4. A group of friends is packing up the red, white, and blue glassware from a Fourth of July celebration. Each glass is a perfect cylinder, two inches in diameter. They have boxes that are a foot wide and 16 inches long, and the same height as the glasses. What is the maximum number of glasses that can be packed into one of the boxes, assuming all of the glasses are standing upright?
5. What country in South America do you hit first if you fly due south out of Key West, FL?
Puzzles courtesy of Martin Gardner, Peter Winkler, and the team at Varsity Math (varsity.momath.org)
Click here to reveal the answers
Answers
1. Maine
2. Place a red and a blue ball on one side, and a red and a white ball on the other.
Case 1. The two sides are the same:
Since there are not four heavy balls or four light balls, each side must have one light and one heavy ball. Thus the heavy red ball has a light ball with it, and the light red ball has a heavy ball with it.
For the second weighing, just compare the two red balls. The heavy and light red balls are directly discovered. Moreover, the ball with the heavy red ball in the first weighing is now known to be light, and the ball with the light red ball in the first weighing is now known to be heavy. The untouched blue and white balls will respectively be the opposite of their known same-colour partners.
Case 2. One side is heavier:
The heavier side cannot have the light red ball, since such a situation would give at most one heavy ball on the heavier side, and at least one heavy (red) ball on the lighter side, which is impossible. So the heavier side must have the heavy red ball, and the lighter side has the light red ball. The red balls are distinguished.
For the second weighing, place the two red balls on one side, and the one blue and one white ball from the first weighing on the other side. If the blue and white side is heavier than the red side, both balls are heavy. If the blue and white side is lighter, both balls are light. If the balance is the same, then one ball is light and the other heavy. The ball that was with the heavy red in the first weighing is heavy, and the ball with the light red in the first weighing is light, since the reverse would have resulted in a tie in the first weighing. As before, the untouched blue and white balls will respectively be the opposite of their known same-color partners.
3. Los Angeles
4. Since the glass height and the box height match and the glasses must be upright, this is really a two-dimensional problem of packing circles into a rectangle. Also, to simplify the numbers slightly, notice that we can divide everything by two: the problem is the same as packing unit-diameter circles into a six-by-eight rectangle. It is tempting therefore to think of placing the circles in a square grid pattern, for a total of 48 circles fitting in the rectangle. On the other hand, it is well known that the overall most efficient packing of circles in the plane is in a hexagonal grid, so we need to check how many circles can fit in the rectangle in a hexagonal grid pattern. Imagine an infinite hexagonal grid of unit-diameter circles; how can a six-by-eight rectangle be placed thereon to maximize the number of circles in the rectangle?
First, note that we can assume that at least one circle must be tangent to a side of the rectangle — if not, consider the circle that comes closest to a side without being tangent to it, and slide the rectangle toward the center of that circle until it becomes tangent. No formerly whole circles are cut off, because if so, they would have been closer to a side. All that might possibly happen is that a previously partial circle may become whole. In any case, the number of circles does not decrease. Similarly, we can assume that at least one side is tangent to more than one circle, because we can rotate the rectangle by the smallest increment that makes one side tangent to two circles. Again, this can’t cut off any formerly whole circles, so the number of circles in the rectangle is no fewer after the rotation.

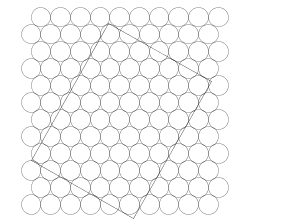 Now it is a matter of trying different configurations in which some side is tangent to at least two circles in a hexagonal packing. A little playing around will make it clear that there are only two orientations that are contenders for packing the most circles — one in which the long side of the rectangle lines up along a row of circles, and one in which the short side of the rectangle lines up along a row of circles. In the former case, we get three rows of 8 circles plus three rows of 7 circles, or 45 circles, into the rectangle — not as good as the square grid. But in the latter case, we get five rows of 6 circles plus four rows of 5 circles for a total of 50 circles, i.e., glasses that the team can pack in a box. (To verify that all 9 rows can fit in the box this way, note that the centers of the rows are the height of a unit equilateral triangle apart, i.e., √3/2, and there is one extra unit of space between the centers of the circles and the walls, and that 1 + 4√3 < 8.)
Now it is a matter of trying different configurations in which some side is tangent to at least two circles in a hexagonal packing. A little playing around will make it clear that there are only two orientations that are contenders for packing the most circles — one in which the long side of the rectangle lines up along a row of circles, and one in which the short side of the rectangle lines up along a row of circles. In the former case, we get three rows of 8 circles plus three rows of 7 circles, or 45 circles, into the rectangle — not as good as the square grid. But in the latter case, we get five rows of 6 circles plus four rows of 5 circles for a total of 50 circles, i.e., glasses that the team can pack in a box. (To verify that all 9 rows can fit in the box this way, note that the centers of the rows are the height of a unit equilateral triangle apart, i.e., √3/2, and there is one extra unit of space between the centers of the circles and the walls, and that 1 + 4√3 < 8.)
5. You pass west of the entire continent.
_____________
Support Join



